はじめに
任意波形発生器 (Arbitrary Waveform Generator: AWG) の代表的なメーカである Keysight の製品には 2 つの DAC を同期させて 1 つの DAC として使う機能がある（sub-DAC, dual-DAC）。この機能の動作モードの中に NRZ (Non-Return-to-Zero), RZ (Return-to-Zero) と Doublet がある [1]。これらの周波数特性は単独の DAC のそれとは異なる。本記事ではその数式を導く。
\[
% general purpose
\newcommand{\ctext}[1]{\raise0.2ex\hbox{\textcircled{\scriptsize{#1}}}}
% mathematics
% general purpose
\DeclarePairedDelimiterX{\parens}[1]{\lparen}{\rparen}{#1}
\DeclarePairedDelimiterX{\braces}[1]{\lbrace}{\rbrace}{#1}
\DeclarePairedDelimiterX{\bracks}[1]{\lbrack}{\rbrack}{#1}
\DeclarePairedDelimiterX{\verts}[1]{|}{|}{#1}
\DeclarePairedDelimiterX{\Verts}[1]{\|}{\|}{#1}
\newcommand{\as}{{\quad\textrm{as}\quad}}
\newcommand{\st}{{\textrm{ s.t. }}}
\DeclarePairedDelimiterX{\setComprehension}[2]{\lbrace}{\rbrace}{#1\,\delimsize\vert\,#2}
\newcommand{\naturalNumbers}{\mathbb{N}}
\newcommand{\integers}{\mathbb{Z}}
\newcommand{\rationalNumbers}{\mathbb{Q}}
\newcommand{\realNumbers}{\mathbb{R}}
\newcommand{\complexNumbers}{\mathbb{C}}
\newcommand{\field}{\mathbb{F}}
\newcommand{\func}[2]{{#1}\parens*{#2}}
\newcommand*{\argmax}{\operatorname*{arg~max}}
\newcommand*{\argmin}{\operatorname*{arg~min}}
% set theory
\newcommand{\range}[2]{\braces*{#1,\dotsc,#2}}
\providecommand{\complement}{}\renewcommand{\complement}{\mathrm{c}}
\newcommand{\ind}[2]{\mathbbm{1}_{#1}\parens*{#2}}
\newcommand{\indII}[1]{\mathbbm{1}\braces*{#1}}
% number theory
\newcommand{\abs}[1]{\verts*{#1}}
\newcommand{\combi}[2]{{_{#1}\mathrm{C}_{#2}}}
\newcommand{\perm}[2]{{_{#1}\mathrm{P}_{#2}}}
\newcommand{\GaloisField}[1]{\mathrm{GF}\parens*{#1}}
% analysis
\newcommand{\NapierE}{\mathrm{e}}
\newcommand{\sgn}[1]{\operatorname{sgn}\parens*{#1}}
\newcommand*{\rect}{\operatorname{rect}}
\newcommand{\cl}[1]{\operatorname{cl}#1}
\newcommand{\Img}[1]{\operatorname{Img}\parens*{#1}}
\newcommand{\dom}[1]{\operatorname{dom}\parens*{#1}}
\newcommand{\norm}[1]{\Verts*{#1}}
\newcommand{\floor}[1]{\left\lfloor#1\right\rfloor}
\newcommand{\ceil}[1]{\left\lceil#1\right\rceil}
\newcommand{\expo}[1]{\exp\parens*{#1}}
\newcommand{\sinc}{\operatorname{sinc}}
\newcommand{\nsinc}{\operatorname{nsinc}}
\newcommand{\GammaFunc}[1]{\Gamma\parens*{#1}}
\newcommand*{\erf}{\operatorname{erf}}
% inverse trigonometric functions
\newcommand{\asin}[1]{\operatorname{Sin}^{-1}{#1}}
\newcommand{\acos}[1]{\operatorname{Cos}^{-1}{#1}}
\newcommand{\atan}[1]{\operatorname{{Tan}^{-1}}{#1}}
\newcommand{\atanEx}[2]{\atan{\parens*{#1,#2}}}
% convolution
\newcommand{\cycConv}[2]{{#1}\underset{\text{cyc}}{*}{#2}}
% derivative
\newcommand{\deriv}[3]{\frac{\operatorname{d}^{#3}#1}{\operatorname{d}{#2}^{#3}}}
\newcommand{\derivLong}[3]{\frac{\operatorname{d}^{#3}}{\operatorname{d}{#2}^{#3}}#1}
\newcommand{\partDeriv}[3]{\frac{\operatorname{\partial}^{#3}#1}{\operatorname{\partial}{#2}^{#3}}}
\newcommand{\partDerivLong}[3]{\frac{\operatorname{\partial}^{#3}}{\operatorname{\partial}{#2}^{#3}}#1}
\newcommand{\partDerivIIHetero}[3]{\frac{\operatorname{\partial}^2#1}{\partial#2\operatorname{\partial}#3}}
\newcommand{\partDerivIIHeteroLong}[3]{{\frac{\operatorname{\partial}^2}{\partial#2\operatorname{\partial}#3}#1}}
% integral
\newcommand{\integrate}[5]{\int_{#1}^{#2}{#3}{\mathrm{d}^{#4}}#5}
\newcommand{\LebInteg}[4]{\int_{#1} {#2} {#3}\parens*{\mathrm{d}#4}}
% complex analysis
\newcommand{\conj}[1]{\overline{#1}}
\providecommand{\Re}{}\renewcommand{\Re}[1]{{\operatorname{Re}{\parens*{#1}}}}
\providecommand{\Im}{}\renewcommand{\Im}[1]{{\operatorname{Im}{\parens*{#1}}}}
\newcommand{\Arg}{\operatorname{Arg}}
\newcommand{\Log}{\operatorname{Log}}
% Laplace transform
\newcommand{\LPLC}[1]{\operatorname{\mathcal{L}}\parens*{#1}}
\newcommand{\ILPLC}[1]{\operatorname{\mathcal{L}}^{-1}\parens*{#1}}
% Discrete Fourier Transform
\newcommand{\DFT}[1]{\mathrm{DFT}\parens*{#1}}
% Z transform
\newcommand{\ZTrans}[1]{\operatorname{\mathcal{Z}}\parens*{#1}}
\newcommand{\IZTrans}[1]{\operatorname{\mathcal{Z}}^{-1}\parens*{#1}}
% linear algebra
\newcommand{\bm}[1]{{\boldsymbol{#1}}}
\newcommand{\matEntry}[3]{#1\bracks*{#2}\bracks*{#3}}
\newcommand{\matPart}[5]{\matEntry{#1}{#2:#3}{#4:#5}}
\newcommand{\diag}[1]{\operatorname{diag}\parens*{#1}}
\newcommand{\tr}[1]{\operatorname{tr}{\parens*{#1}}}
\newcommand{\inprod}[2]{\left\langle#1,#2\right\rangle}
\newcommand{\HadamardProd}{\odot}
\newcommand{\HadamardDiv}{\oslash}
\newcommand{\Span}[1]{\operatorname{span}\bracks*{#1}}
\newcommand{\Ker}[1]{\operatorname{Ker}\parens*{#1}}
\newcommand{\rank}[1]{\operatorname{rank}\parens*{#1}}
% vector
% unit vector
\newcommand{\vix}{\bm{i}_x}
\newcommand{\viy}{\bm{i}_y}
\newcommand{\viz}{\bm{i}_z}
% probability theory
\newcommand{\PDF}[2]{\operatorname{PDF}\bracks*{#1,\;#2}}
\newcommand{\Ber}[1]{\operatorname{Ber}\parens*{#1}}
\newcommand{\Beta}[2]{\operatorname{Beta}\parens*{#1,#2}}
\newcommand{\ExpDist}[1]{\operatorname{ExpDist}\parens*{#1}}
\newcommand{\ErlangDist}[2]{\operatorname{ErlangDist}\parens*{#1,#2}}
\newcommand{\PoissonDist}[1]{\operatorname{PoissonDist}\parens*{#1}}
\newcommand{\GammaDist}[2]{\operatorname{Gamma}\parens*{#1,#2}}
\newcommand{\cind}[2]{\ind{#1\left| #2\right.}} % conditional indicator function
\providecommand{\Pr}{}\renewcommand{\Pr}[1]{\operatorname{Pr}\parens*{#1}}
\DeclarePairedDelimiterX{\cPrParens}[2]{(}{)}{#1\,\delimsize\vert\,#2}
\newcommand{\cPr}[2]{\operatorname{Pr}\cPrParens{#1}{#2}}
\newcommand{\E}[2]{\operatorname{E}_{#1}\bracks*{#2}}
\newcommand{\cE}[3]{\E{#1}{\left.#2\right|#3}}
\newcommand{\Var}[2]{\operatorname{Var}_{#1}\bracks*{#2}}
\newcommand{\Cov}[2]{\operatorname{Cov}\bracks*{#1,#2}}
\newcommand{\CovMat}[1]{\operatorname{Cov}\bracks*{#1}}
% graph theory
\newcommand{\neighborhood}{\mathcal{N}}
% programming
\newcommand{\plpl}{\mathrel{++}}
\newcommand{\pleq}{\mathrel{+}=}
\newcommand{\asteq}{\mathrel{*}=}
\]
\[
\newcommand{\Ts}{T_\text{s}}
\newcommand{\xd}{x_\text{d}}
\newcommand{\Xd}{X_\text{d}}
\newcommand{\xNRZ}{x_\text{NRZ}}
\newcommand{\xRZ}{x_\text{RZ}}
\newcommand{\xDbl}{x_\text{Dbl}}
\newcommand{\XNRZ}{X_\text{NRZ}}
\newcommand{\XRZ}{X_\text{RZ}}
\newcommand{\XDbl}{X_\text{Dbl}}
\]
主張
次の通り記号を定める。応用性を重視し全て無次元量とする。実用にあたっては適当に計量単位を選べばよい。
- $\Ts>0$：sub-DAC のサンプリング周期
- $\xd:\integers\to\realNumbers$：dual-DAC に送られる離散時間信号
- $\Xd:\realNumbers\to\complexNumbers$：$\xd$ の周波数表示された DTFT
- $\xNRZ,\;\xRZ,\;\xDbl:\integers\to\realNumbers$：それぞれ NRZ, RZ, Doublet モードに於ける dual-DAC の出力
- $\XNRZ,\;\XRZ,\;\XDbl:\realNumbers\to\complexNumbers$：それぞれ NRZ, RZ, Doublet モードに於ける dual-DAC の周波数表示された Fourier 変換
上記の設定の下に次式が成り立つ。
\[
\begin{align*}
\XNRZ(f) &= \Ts\exp(-i\pi f\Ts)\sinc(\pi f\Ts)\Xd(f) \\
\XRZ(f) &= \frac{\Ts}{2}\exp\parens*{-i\frac{\pi}{2}f\Ts}\sinc\parens*{\frac{\pi}{2}f\Ts}\Xd(f) \\
\XDbl(f) &= i\Ts\exp(-i\pi f\Ts)\sin\parens*{\frac{\pi}{2}f\Ts}\sinc\parens*{\frac{\pi}{2}f\Ts}\Xd(f)
\end{align*}
\]
次の図は上の 3 つの式の $\Xd(f)$ を除いた部分の絶対値である。sub-DAC の 1st, 2nd Nyquist 領域が半透明の矩形で示されている。
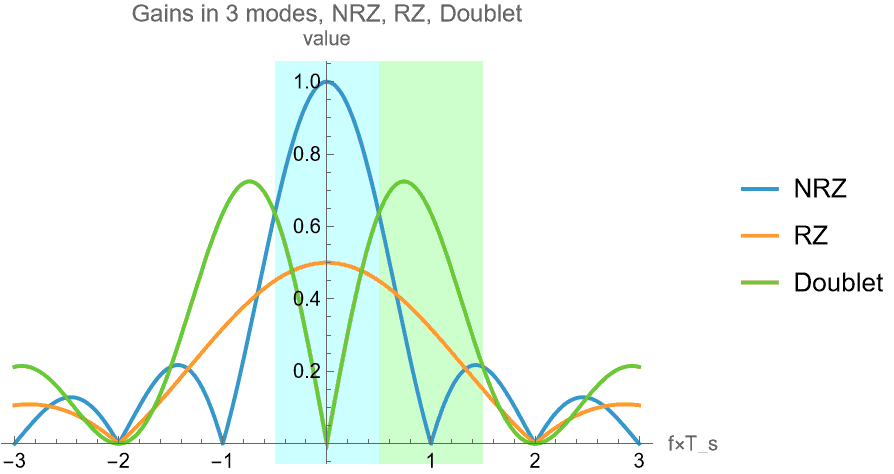NRZ, RZ, Doublet のゲイン特性
NRZ の特性は普通の aperture 効果と等しい。RZ では全体的なゲインが NRZ と比べて 1/2 になるが 2nd Nyquist 領域に null が無い。Doublet では DC に null があるが 2nd Nyquist 領域でゲインが最大になる。
導出
\[
\newcommand{\uRZ}{u_\text{RZ}}
\]
NRZ については [2] 「14.1.1 0 次ホールドされた離散時間信号の周波数スペクトラム」に述べられているので省略する。
RZ
$\xRZ$ は次のように表される。
\[ \xRZ(t) = \sum_{n=-\infty}^\infty \xd(n)\uRZ(t-n\Ts) \]
ここに $\uRZ$ は次式で表される矩形パルスである。
\[
\uRZ(t) = \begin{cases}
1, & 0 \leq t < \Ts/2 \\
0, & \text{otherwise}
\end{cases}
\]
これを用いて $\XRZ$ を求める。
\[
\begin{align*}
\XRZ(f) &= \integrate{-\infty}{\infty}{\xRZ(t)\exp(-i2\pi ft)}{}{t} = \sum_{n=-\infty}^\infty \xd(n)\integrate{-\infty}{\infty}{\uRZ(t-n\Ts)\exp(-i2\pi ft)}{}{t} \\
&= \sum_{n=-\infty}^\infty \xd(n)\underbrace{\integrate{n\Ts}{n\Ts+\Ts/2}{\uRZ(t-n\Ts)\exp(-i2\pi ft)}{}{t}}_{(1)} \\
(1) &= \frac{1}{i2\pi f}\exp(-i2\pi f n\Ts)\bracks{1-\exp(-i\pi f\Ts)} \\
&= \frac{1}{i2\pi f}\exp(-i2\pi f n\Ts)\exp\parens*{-i\frac{\pi}{2}f\Ts}\bracks*{\exp\parens*{i\frac{\pi}{2}f\Ts}-\exp\parens*{-i\frac{\pi}{2}f\Ts}} \\
&= \frac{\Ts}{2}\exp\parens*{-i\frac{\pi}{2}f\Ts}\sinc\parens*{\frac{\pi}{2}f\Ts}\exp(-i2\pi f n\Ts) \\
\therefore \XRZ(f) &= \frac{\Ts}{2}\exp\parens*{-i\frac{\pi}{2}f\Ts}\sinc\parens*{\frac{\pi}{2}f\Ts}\sum_{n=-\infty}^\infty \xd(n)\exp(-i2\pi f n\Ts) \\
&= \frac{\Ts}{2}\exp\parens*{-i\frac{\pi}{2}f\Ts}\sinc\parens*{\frac{\pi}{2}f\Ts}\Xd(f)
\end{align*}
\]
Doublet
$\xDbl(t) = \xRZ(t) – \xRZ(t-\Ts/2)$ である。時間シフトと Fourier 変換の関係から次式が成り立つ。
\[
\begin{align*}
\XDbl(f) &= \XRZ(f)\parens{1-\exp(-i\pi f\Ts)} = 2i\exp\parens*{-i\frac{\pi}{2}f\Ts}\sin\parens*{\frac{\pi}{2}f\Ts}\XRZ(f) \\
&= i\Ts\exp(-i\pi f\Ts)\sin\parens*{\frac{\pi}{2}f\Ts}\sinc\parens*{\frac{\pi}{2}f\Ts}\Xd(f)
\end{align*}
\]
具体例
Mathematica による計算例を示す。
参考文献
- Fundamentals of Arbitrary Waveform Generation, AWG Primer – Reference Guide
- Signal-Processing-Memorandum v0.15.0
